Das Fachgebiet GNSS-Fernerkundung, Navigation und Positionierung hat folgende Forschungsschwerpunkte:
- Entwicklung und Verbesserung wissenschaftlicher GNSS-Auswertesoftware für Erdbeobachtungsanwendungen mit den Schwerpunkten Atmosphärensondierung und Fernerkundung von Wasser-, Eis- und Landoberflächen,
- Operationelle Verarbeitung von satelliten- und bodengestützten GNSS-Atmosphärensondierungsdaten und Bereitstellung für Wetterdienste,
- Entwicklung und Anwendung von GNSS-Software unter Echtzeitaspekten, z.B. für die Nutzung in Frühwarnsystemen und zur Atmosphärensondierung,
- Multidisziplinäre Anwendungen von GNSS-Erdbeobachtungsdaten vor allem in den Geowissenschaften und zur Umweltüberwachung,
- Initiierung neuer Satellitenmissionen mit GNSS-Komponenten zur präzisen Bahnbestimmung, Atmosphärensondierung und Reflektometrie,
- Entwicklung von spezifischen wissenschaftlichen GNSS-Empfängern für GNSS-Erdbeobachtungsanwendungen.
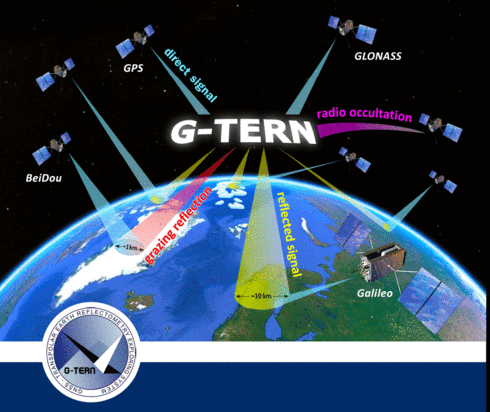
Ein Höhepunkt der wissenschaftlichen Aktivitäten im Fachgebiet war eine neuartige Satellitenmission zur Klimaforschung mit Fokus auf die Polarregionen. Dieses Experiment wurde im Rahmen der Earth Explorer 9 Ausschreibung der Europäischen Weltraumbehörde ESA in einem großen internationalen Team von Wissenschaftlern und Ingenieuren unter gemeinsamer Leitung von IEEC Barcelona und TUB/GFZ vorgeschlagen: G-TERN.
Standort
Kontakt
| Einrichtung | GNSS-Fernerkundung, Navigation und Positionierung |
|---|---|
| Sekretariat | KAI 2-2 |
| Gebäude | KAI |
| Raum | KAI 2223 |
